From a legend told by an old alloy Ti-6-4 who survived a severe plastic deformation to a young inexperienced alloy.
Below are some of my contributions in this area.
Proposing a continuum model of grain refinement and damage of polycrystalline materials under severe plastic deformation (SPD). The model makes two natural assumptions: (1) the self-similarity of grain refinement and micro-damage, and (2) the complementarity of these two processes. The model uses a system of kinetic equations to explain the formation of structure during an SPD process. Analyzing these equations gives the following conclusions:
- The intensity of grain refinement increases with the pressure in the center of deformation. Fracture, on the other hand, decreases as the pressure increases.
- For any metal, an ultra-fine grained (UFG) structure is obtained faster by the processes that lead to a larger decrease in ductility of this metal under the zero level of hydrostatic pressure in the center of deformation. In order to obtain a UFG structure, it is necessary to carry out these processes under high hydrostatic pressure in the center of deformation. In this case, the relaxation of internal stresses occurs along the direction of crystalline fragmentation instead of micropore formation.
- According to the above criterion, simple shear should lead to a more intensive grain refinement than does pure shear, when deformation is performed under high pressure.
- Quasi-monotone deformations provide higher grain refinement intensity than do cyclic deformations. In order to increase the intensity of grain refinement under cyclic deformation, one has to increase the amplitude of deformation. Cyclic deformations provide higher ductility than quasi-monotone deformations at the same equivalent strain.
- A metal becomes more ductile after it undergoes a large deformation under high pressure.
Y. Beygelzimer. Grain refinement versus voids accumulation during severe plastic deformations of polycrystals: A mathematical simulation, Mechanics of Materials, Vol. 37, No. 7, pp. 753--767 (2005).
Grain Refinement and Viscous Fracture of Metals during Severe Plastic Deformation: Mathematical Simulation (ppt, pdf), 2004.
Developing a continuum theory of plastic deformation in structurally-inhomogeneous porous bodies. Based on this theory, proposing a mathematical model of metal forming of porous and powder materials. Developing a new model for predicting the ductility of materials under deformation.
Y. Beygelzimer, V. Varyukhin, B. Efros. Physical mechanics of hydrostatic treatment of materials. Donetsk: DonFTI NANU, 192 pages, 2000 (in Russian).
Y. Beygelzimer. Constitutive Equations of a Porous Body with a Structurally-Inhomogeneous Matrix, Proceedings of International Workshop on Modelling of Metal Powder Forming Processes, Grenoble, France, July 21--23, 1997, pp. 57--67.
Y. Beygelzimer, B. Efros, V. Varyukhin, A. Khokhlov. A continuum model of a structurally-inhomogeneous porous body and its application for the study of stability and viscous fracture of materials deformed under pressure. Engineering Fracture Mechanics, Vol. 48, No. 5, pp. 629--640 (1994).
On some models in metal forming: From continuum mechanics to mirco-mechanics and back (ppt, pdf), 2002.
Proposing a computational model of elastic-plastic deformation of micro-inhomogeneous materials, which accounts for rotations of structural elements. Introducing new geometrical structures into plasticity theory ("thick yield surface", "cloud of internal stress") which effectively describe elastic-plastic deformation of micro-inhomogeneous materials. The picture below demonstrates a cloud of internal stress.
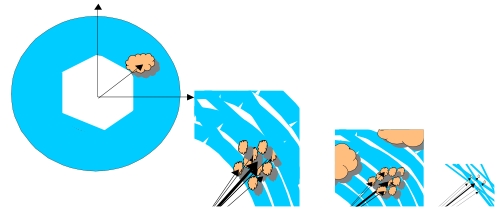
Publications:
Y. Beygelzimer, A. Spuskanyuk. The thick yield surface: An idea and approach for investigating its structure. Philosophical Magazine A, Vol. 79, No. 10, pp. 2437--2459 (1999).
Y. Beygelzimer, A. Spuskanyuk, V. Varyukhin. On The Loading Surface of Microinhomogeneous Materials, Resent Development in Computer Modeling of Powder Metallurgy Processes, IOS Press, pp. 17--28, 2001.
